Time-Weighted Return (TWRR) Calculator
The Time-Weighted Return is 0.00%
The investment duration is 1 day. Therefore, the annualized rate of return is 0.00%.
0.000.00What is Time-Weighted Rate of Return (TWR)?
The Time-Weighted Rate of Return (TWR or TWRR) is a method of calculating the performance of an investment portfolio over time. It is a measure that eliminates the impact of external cash flows into or out of the portfolio, which can distort the true performance of the investments.
This method is particularly useful for evaluating the performance of investment managers and portfolios in situations where there are significant cash inflows or outflows, such as in the case of institutional portfolios or mutual funds. It allows investors to assess how well the investment decisions contributed to the portfolio's growth, independent of the timing and size of cash flows.
Time-Weighted Rate of Return (TWR) Formula
The formula for calculating the time-weighted rate of return is as follows:
Where each Pn represents the return an individual investment period. The formula to calculate the return for each investment period Pn is:
And, where n is the number of investment periods.
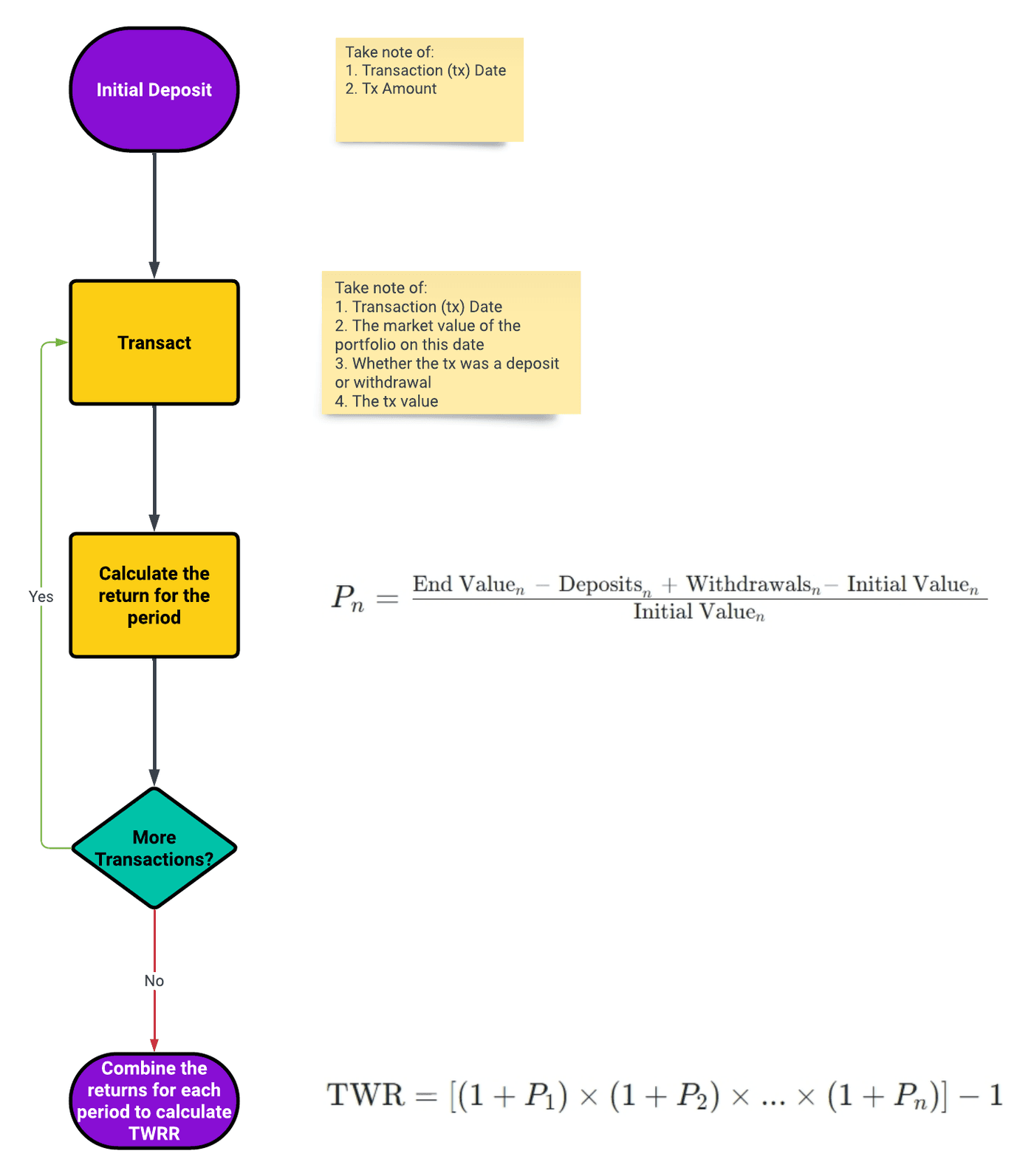
How to Calculate Time-Weighted Rate of Return
- Structure the data: your data set will consist of an initial deposit and a collection of investment periods following that. Each period starts at the date of the last transaction (deposit or withdrawal or both) and ends at the date of the next transaction.
- Calculate the return for each period n by using the formula . Then, add 1 to the calculated rate of return for each period i.e. (1 + Pn)
- Multiply all of the calculated period return values together. Subtract 1 from the total to complete the calculation of the TWR. In other words you will have calculated .
Here's the calculation process represented as a flowchart:
Example: Calculating the Time-Weighted Return of a Portfolio
Calculating TWR can be intimidating at first so let's work through an example. You can load the example into the calculator by clicking the Load Example button above.
1. Structure the Data
The initial deposit details:
| Date | Value |
|---|---|
| 31 Dec 2019 | 1000 |
There are 4 transactions over the lifetime of the investment:
| From | To | Initial Value | End Value | Deposit | Withdraw | Balance |
|---|---|---|---|---|---|---|
| 31 Dec 2019 | 30 Jun 2020 | 1000 | 1200 | 100 | 0 | 1300 |
| 30 Jun 2020 | 31 Dec 2020 | 1300 | 1170 | 100 | 50 | 1220 |
| 31 Dec 2020 | 30 Jun 2021 | 1220 | 1403 | 100 | 0 | 1503 |
| 30 Jun 2021 | 31 Dec 2021 | 1503 | 1653.3 | 100 | 50 | 1703.3 |
2. Calculate the Return for Each Period
| Period | Formula | Calculated Result | 1 + Pn |
|---|---|---|---|
| P1 | (1300 - 100 + 0 - 1000) ÷ 1000 | 0.20 (20.00%) | 1.2 |
| P2 | (1220 - 100 + 50 - 1300) ÷ 1300 | -0.10 (-10.00%) | 0.9 |
| P3 | (1503 - 100 + 0 - 1220) ÷ 1220 | 0.15 (15.00%) | 1.15 |
| P4 | (1703.3 - 100 + 50 - 1503) ÷ 1503 | 0.10 (10.00%) | 1.1 |
3. Multiply the Returns
Multiple each value from the 1 + Pn column above. Subtract 1 from the resultant product to calculate the time-weighted rate of return i.e.
TWR = [(1 + 0.20) × (1 + -0.10) × (1 + 0.15) × (1 + 0.10)] - 1 = 0.36619999999999986 ≈ 36.62%
Why Use the Time-Weighted Rate of Return?
The Time-Weighted Rate of Return provides a measure of how well an investment portfolio has performed over a specific period, while mitigating the impact of external cash flows. Here are some key reasons to use TWRR.
- Pure Investment Performance: TWR isolates the performance of the underlying investments from the effects of external contributions or withdrawals. This makes it a useful metric for assessing the skill of fund managers and the performance of investment decisions.
- Comparability: TWR allows for the comparison of the performance of different portfolios or investment managers.
- Elimination of Timing Bias: By ignoring the impact of cash flows, TWR eliminates timing biases that can distort other performance metrics. This is particularly important in the evaluation of portfolios with significant cash inflows or outflows.
- Accurate Reflection of Investment Strategy: TWR provides a more accurate reflection of the investment strategy's success, as it measures the compound growth rate of the portfolio, adjusting for the effects of compounding over multiple sub-periods.
- Useful for Institutional Investors: Institutional investors, such as pension funds and endowments, often deal with substantial cash flows. TWR is valuable in such scenarios because it assesses investment performance independently of these cash movements.
- Periodic Assessment: TWR can be calculated for different sub-periods within the overall evaluation period, allowing investors to assess performance over specific time intervals.w
Limitations of the Time-Weighted Rate of Return
While the Time-Weighted Rate of Return (TWR) is a widely used and valuable performance metric, it does have certain limitations and considerations that investors should be aware of:
- Ignores the Impact of Cash Flows: One of the main limitations is that TWR completely ignores the impact of cash inflows and outflows. While this is an advantage for isolating investment performance, it can be a drawback if the investor is interested in understanding the actual return experienced, especially in situations with significant cash movements.
- Assumes Reinvestment of Cash Flows: TWR assumes that any cash inflows are reinvested in the portfolio at the beginning of each sub-period. This assumption may not reflect the actual behavior of investors who might hold cash in money market instruments or other assets. May Overstate Performance in Presence of Large Cash Flows: In situations where there are substantial cash flows, TWR may overstate or understate the performance of the underlying investments. This is because the compounding effect of large cash flows may not align with the investor's actual experience.
- Not Ideal for Personalized Performance Measurement: TWR is more suitable for institutional investors and fund managers, and it might not be the best metric for individual investors who have different investment goals and cash flow patterns.
- Sensitive to Sub-Period Selection: The choice of sub-periods for TWR calculation can impact the results. Different sub-periods may yield different TWR values, and the metric may not capture the performance accurately if the chosen sub-periods are not representative of the investor's experience.
- Requires Regular Valuation Data: TWR calculations require accurate and regular valuation data for the portfolio. In cases where frequent and reliable valuation data is not available, TWR may be challenging to calculate.
- Assumes Rebalancing of Portfolio: TWR assumes that the portfolio is rebalanced at the beginning of each sub-period. This might not be the case in reality, especially for portfolios with a long-term investment horizon and infrequent rebalancing.
- May Not Reflect Investor Behavior: TWR focuses on the performance of the underlying investments, but it may not capture the behavioral aspects of investors, such as market timing or emotional decision-making.
In summary, while TWR is a valuable tool for assessing the investment performance of portfolios, it is essential to consider its limitations and, when necessary, complement it with other performance metrics, such as the Money-Weighted Rate of Return (MWRR), that account for the impact of cash flows on the investor's actual return.
Alternatives to Time-Weighted Rate of Return
Several alternative performance metrics exist, each with its own strengths and weaknesses. The choice of metric depends on the specific goals and circumstances of the investor. Here are some common alternatives to the Time-Weighted Rate of Return (TWR):
- Money-Weighted Rate of Return (MWRR or IRR): Unlike TWR, MWRR accounts for the impact of cash inflows and outflows. It reflects the actual rate of return experienced by the investor by considering the timing and size of cash flows. MWRR is suitable for individual investors who want to measure the performance of their entire portfolio, including the effects of their personal contributions and withdrawals.
- Geometric Mean Return: Geometric mean return is another method for calculating the compound rate of return. It considers the compounding effect of returns over multiple periods, providing a single average rate of return. However, it does not explicitly account for the impact of cash flows.